Questions

https://docs.google.com/forms/d/e/1FAIpQLSfFIeDq6mg117SAuGWmrUKP-b0xFTSr3YmqZ8s3Is9-WKpR1A/viewform


http://www.mathopolis.com/questions/index.php

Video

Part 1

Part 2

Multiplication Video

https://fantasticmathworld.blogspot.my/2017/09/video.html

Games

LET HAVE SOME FUN ↓↓↓

Level 1

Level 2

Level 3

Level 4

Class Dicussion

discussion clasa

Dicussion

Dicussion

Questions

Individual Questions

Questions

Level 1

Sketch trigonometric graph

Plot of Sine

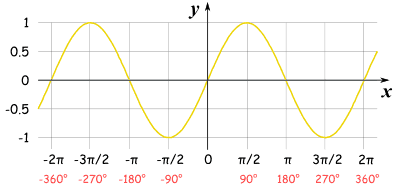
The Sine Function has this beautiful up-down curve (which repeats every 2π radians, or 360°).

It starts at 0, heads up to 1 by π/2 radians (90°) and then heads down to -1.

Plot of Cosine

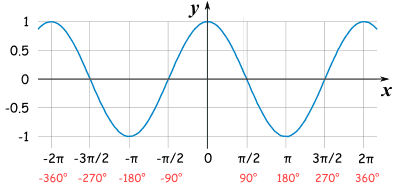
Cosine is just like Sine, but it starts at 1 and heads down until π radians (180°) and then heads up again.

Plot of Sine and Cosine

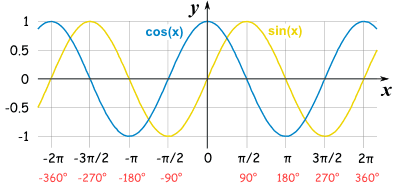
In fact Sine and Cosine are like good friends: they follow each other, exactly "π/2" radians, or 90°, apart.

Plot of the Tangent Function

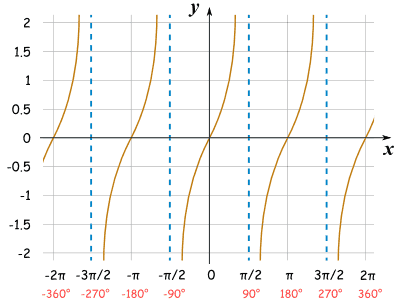
The Tangent function has a completely different shape ... it goes between negative and positive Infinity, crossing through 0 (every π radians, or 180°), as shown on this plot.

At π/2 radians, or 90° (and -π/2, 3π/2, etc) the function is officially undefined, because it could be positive Infinity or negative Infinity.

Inverse Sine, Cosine and Tangent

The Inverse Sine, Cosine and Tangent graphs are:

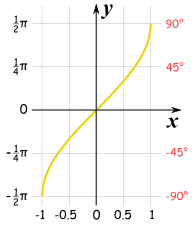
Inverse Sine

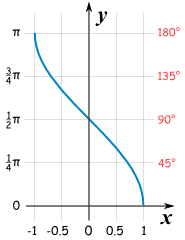
Inverse Cosine

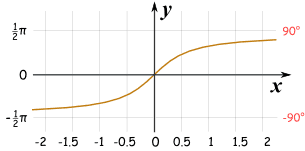
Inverse Tangent

Mirror Images

Here is Cosine and Inverse Cosine plotted on the same graph:

Cosine and Inverse Cosine

They are mirror images (about the diagonal)!

The same is true for Sine and Inverse Sine and for Tangent and Inverse Tangent. Can you see this in the graphs above?

Empirical or Statistical Probability

Empirical Probability Formula

Empirical probability is one of the objective probabilities. It is the relative frequency of the occurrence of an event and is determined by actual observation of an experiment. Consider an experiment, event A occurs "f" number of times if we repeat experiment n times.  

Then fn$\frac{f}{n}$ is known as frequency ratio.  The particular limiting value from the frequency ratio as the number of repetitions becomes infinitely large is known as probability of the given event.

 Probability is divided into two parts: theoretical probability and empirical probability. Sometimes empirical probability named as experimental probability. The probability of an event will always be a number between zero and one. An empirical probability of zero (0) means that the event never occurred and one (1) means that the event always occurred. In this section will study about empirical probability formula in detail.

Formula

Empirical probability of an event is estimate that the event will occur based on sample data of performing repeated trials of a probability experiment. The empirical approach to probability is based on law of large numbers. So to achieve more accuracy in the result, collect more observations which provide more accurate estimate of the probability.

Empirical probability formula = Probability of an event happening is the ratio of the time similar events happened in the past.

or Empirical probability = Number of times event occursTotal number of times experiment performed$\frac{Numberoftimeseventoccurs}{Totalnumberoftimesexperimentperformed}$

Examples

Below are few examples on empirical probability.

Example 1: Eight coins are tossed. Below are frequencies of the number of tails are appeared.

X	0	1	2	3	4	5	6	7	8
F	2	15	29	57	70	59	20	10	8

Calculate the probability of occurred tails:

i) Equal to 5  

ii) Less than 4

iii) More than 6

Solution: 

Total number of heads occurred in an experiment = 2 + 15 + 29 + 57 + 70 + 59 + 20 + 10 + 8 = 270

i) P( occurred tails equal to 5). 

From given table, number of required cases = 59

Therefore P( tails equal to 5) = 59270$\frac{59}{270}$ = 0.22

ii)  P(occurred tails less than 4)

From given table, number of favorable cases = 2 + 15 + 29 + 57 = 103

Therefore P(tails less than 4) = 103270$\frac{103}{270}$ = 0.38
iii) P( more than 6 tails) 
Total number of cases = 10 + 8 = 18
So P(tails more than 6) = 18270$\frac{18}{270}$ = 0.07

Example 2: The following are the marks obtained by 1200 students in a particular examination.

Marks	100-120	120-140	140-160	160-180	180-200
No. of Students	63	142	500	320	175

Find the probability that a student selected has marks :

i) Under 140

ii) Above 180

iii) Between 140 and 200

Solution: 

Total marks obtained by students = 63 + 142 + 500 + 320 + 175 = 1200

i) Probability that a selected student get marks under 140.

There are 63 + 142 = 205 students.

∴$\therefore$ P(student scored marks under 140)= 2051200$\frac{205}{1200}$ = 0.17
ii) Probability that a selected student get marks above 180.

There are 175 students.

∴$\therefore$ P(student scored marks above 180) = 1751200$\frac{175}{1200}$ = 0.15
iii) Probability that a selected student get marks between 140 and 200.

There are 500 + 320 + 175 = 995 students.

∴$\therefore$ P(student scored marks between 140 and 200) = 9951200$\frac{995}{1200}$ = 0.83

Example 3: A coin is thrown 100 times out of which head appears 12 times. Find the experimental probability of getting the head?

Solution:

The coin is thrown 100 times. So total number of trails =100

Given head occurs 12 times. So the number of times the required event occurs = 12

Therefore probability of getting the event of head = 12100$\frac{12}{100}$ = 0.12 (Using experimental probability formula)